Would This Get Credit? AP Statistics Exam 2024 #5
- Chris Viste

- Mar 10
- 11 min read
Updated: Mar 11

This blog post was written by Chris Viste, who was a Table Leader for Question 5. Chris Viste is in her 30th year teaching math at New Berlin West High School in New Berlin, Wisconsin. She has been participating in the AP Statistics Reading for twelve years and has been a Table Leader and an Early Table Leader. In addition to teaching AP Stats, Chris also teaches AP Calc BC, Algebra 2, Statistics and Functions & Trig. She is the advisor for the Math Team and The Way (a Christian student group). Chris's dream job is to be a statistician for the Milwaukee Brewers.
This is the multi-focus free-response question on the exam that incorporates both probability and inference. The probability parts focused on calculating a joint probability and a conditional probability and the inference parts focused on identifying the correct inference procedure, stating hypotheses, and writing a conclusion given a p-value.
Baseball cards are trading cards that feature data on a player’s performance in baseball games. Michelle is at a national baseball card collector’s convention with approximately 20,000 attendees. She notices that some collectors have both regular cards, which are easily obtained, and rare cards, which are harder to obtain. Michelle believes that there is a relationship between the number of months a collector has been collecting baseball cards and whether the majority of the cards (cards appearing more often) in their collection are regular or rare. She obtains information from a random sample of 500 baseball card collectors at the convention and records how many full months they have been collecting baseball cards and whether the majority of the cards in their card collection are regular or rare. Her results are displayed in a two-way table.

Part (a)
(a) If one collector from the sample is selected at random, what is the probability that the collector has been collecting baseball cards for 11 or more months and has a majority of regular baseball cards? Show your work.
WOULD THIS GET CREDIT?
Response 1:
0.518
Response 2:

Response 3:
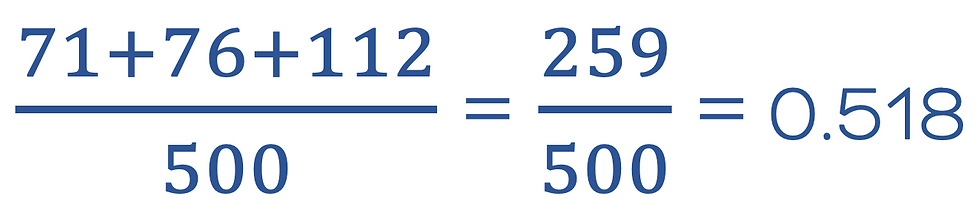
Response 4:
71, 76, and 112 are clearly indicated in the table and 259/500
Response 5:
0.846 + 0.618 - 0.946 = 0.518
Response 6

Response 7:
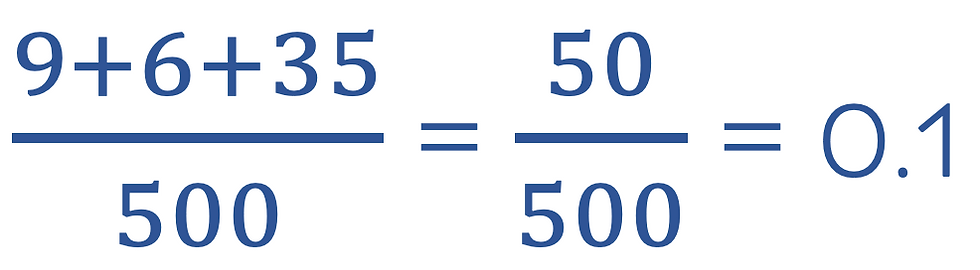
In order to earn full credit in part (a), the response must provide the correct joint probability of 259/500 or 0.518 with supporting work demonstrating how the numerator was computed. (Minor arithmetic or transcription errors are not penalized if work is shown).
Responses 1 and 2 both receive partial credit because no supporting work demonstrating how the numerator is calculated is shown. The probability can be given as a fraction, decimal, or percent.
Response 3 is given full credit because correct supporting work is shown for calculating the numerator along with the correct probability.
Response 4 is given full credit because the response shows supporting work indicating what numbers were added together to get the numerator by marking 71, 76 and 112 in the table and states the correct probability.
Response 5 is given full credit because the response shows work using the formula for the union of two events to find the joint probability by taking P(majority of regular cards) + P(collecting for 11+ months) – P(majority of regular cards OR collecting for 11+ months) and states the correct probability.
Response 6 is given full credit because the response shows work using the general multiplication rule for dependent events to find the joint probability by taking P(majority of regular cards) • P(collecting for 11+ months given|majority of regular cards), and states the correct probability.
Response 7 is given partial credit because the response demonstrates understanding of how to calculate a joint probability but calculated the probability of 11+ months and majority rare cards instead, showing supporting work and the correct probability for this event.
Teaching tips:
Require that students show their work when doing probability calculations. “Bald” answers rarely receive full credit on the AP exam.
Encourage students to write a probability statement for what they’re calculating. While not required, it demonstrates good statistical communication and can be seen as a positive when holistic scoring is required.
Remind students to read the question carefully so they calculate the probability of the correct event.
Part (b)
(b) Given that a randomly selected collector from the sample has been collecting baseball cards for fewer than 6 months, what is the probability the collector has a majority of regular baseball cards? Show your work.
WOULD THIS GET CREDIT?
Response 1:
0.879
Response 2:
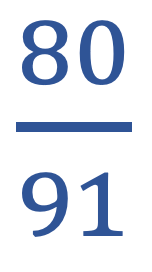
Response 3:
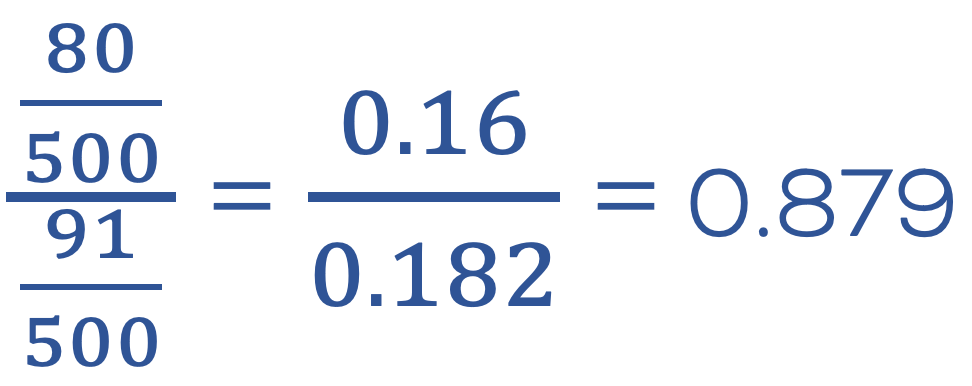
Response 4:
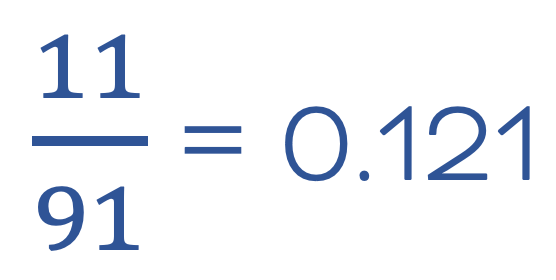
In order to earn full credit for part (b), the response must provide the correct conditional probability of 80/91 or 0.879 with supporting work. (Minor arithmetic or transcription errors are not penalized if work is shown).
Response 1 is given partial credit because it states the correct probability but does not provide supporting work.
Response 2 is given full credit because it states the correct probability and provides sufficient supporting work by using the number of outcomes in the joint event divided by the number of outcomes in the given event. The probability can be given as a fraction, decimal, or percent.
Response 3 is given full credit because it states the correct probability and provides sufficient supporting work by dividing the probability of the joint event by the probability of the given event.
Response 4 is given partial credit because the response demonstrates understanding of how to calculate a conditional probability but calculated the probability of majority rare cards given fewer than 6 months instead, and stated the correct probability for that event.
Teaching tips:
Require that students show their work when doing probability calculations. “Bald” answers rarely receive full credit on the AP exam.
Encourage students to write a probability statement for what they’re calculating. While not required, it demonstrates good statistical communication and can be seen as a positive when holistic scoring is required.
Remind student to read the question carefully so they calculate the probability of the correct event.
Part (c)
(c) Michelle believes there is a relationship between the number of months spent collecting baseball cards and which type of card is the majority in the collection (regular or rare).
(i) Name the hypothesis test Michelle should use to investigate her belief. Do not perform the hypothesis test.
(ii) State the appropriate null and alternative hypotheses for the hypothesis test you identified in (c-i). Do not perform the hypothesis test.
WOULD THIS GET CREDIT?
Response 1:
χ2 Test.
H0: Number of months collecting baseball cards does not affect type of card that is a majority. Ha: Number of months collecting baseball cards does affect type of card that is a majority.
Response 2:
Chi-Square Test of Association.
H0: There is no association between number of months collecting and type of card that is the majority.
Ha: There is an association.
Response 3:
Chi-Square Goodness of Fit Test (or Chi-Square Test of Homogeneity).
H0: There is an association between number of months collecting and type of card that is the majority.
Ha: There is no association between number of months collecting and type of card that is the majority.
Response 4:
Linear Regression T-Test.
H0: There is no correlation between number of months collecting and type of card that is the majority.
Ha: There is a correlation between number of months collecting and type of card that is the majority.
Response 5:
χ2 Test of independence.
H0: Months collecting baseball cards and type of card that is a majority are independent.
Ha: Months collecting baseball cards and type of card that is a majority are not independent.
In order to earn full credit for part (c), the response must indicate a Chi-Square test of association or independence for part (i), and for part (ii) state a null hypothesis of “there is no association between months collecting baseball cards and majority card status,” and an alternative hypothesis of “there is an association between months collecting baseball cards and majority card status.”
Response 1 is given no credit because the name of the test did not specify which Chi-Square test should be used, and even though the hypotheses includes proper context, the response suggests a causal relationship which is not appropriate in this context.
Response 2 is given full credit because the response states a correct name of the test and states correct hypotheses with sufficient context in at least one of the hypotheses.
Response 3 is given no credit because the response states an incorrect name of the test (one that would include only one variable or multiple samples) and reverses the hypotheses.
Response 4 is given no credit because the response states an incorrect name of the test and incorrect hypotheses in context. The term “correlation” should only be used in the case of quantitative variables.
Response 5 is given full credit because the response states a correct name of the test and states correct hypotheses in context.
Teaching tips:
Be specific when stating the type of chi-square test to be used.
Help students understand the differences between the three types of chi-square tests.
Understand that the null hypotheses are statements of “no difference” or “no relationship” and that alternative hypotheses are statements that would typically involve follow-up or taking action, so that hypotheses aren’t stated backwards
Practice writing hypotheses using full context of the problem.
Part (d)
(d) After completing the hypothesis test described in part (c), Michelle obtains a p-value of 0.0075. Assuming the conditions for inference are met, what conclusion should Michelle make about her belief? Justify your response.
WOULD THIS GET CREDIT?
Response 1:
With a p-value of 0.0075, we reject H0. There is an association between the number of months collecting baseball cards and which type of card is the majority in the collection (regular or rare).
Response 2:
Because the p-value is less than 0.01, reject H0. There is not convincing evidence that there is an association between the number of months collecting baseball cards and which type of card is the majority in the collection (regular or rare).
Response 3:
Because 0.0075 < 0.05, Michelle does have convincing evidence that there is an association between the number of months collecting baseball cards and which type of card is the majority in the collection (regular or rare).
Response 4:
With a p-value of 0.0075 and a significance level of 0.05, there is convincing evidence that there is no association between the number of months collecting baseball cards and which type of card is the majority in the collection (regular or rare).
Response 5:
Because the p-value is very small, reject the null hypothesis. There is enough evidence to prove that there is an association between the number of months collecting baseball cards and which type of card is the majority in the collection (regular or rare).
Response 6:
Because 0.0075 is smaller than any reasonable alpha, we reject the null hypotheses that there is not an association between the number of months collecting baseball cards and which type of card is the majority in the collection (regular or rare).
Response 7:
Because 0.0075 < 0.05, reject H0.
Response 8:
Because the p-value is less than alpha, there is sufficient evidence that the number of months collecting baseball cards and which type of card is the majority are not independent.
In order to earn full credit for part (d), the response must provide correct comparison of the p-value to alpha, provide a correct decision about the null and/or alternative hypothesis, and state a conclusion, in context, consistent with and in terms of the stated alternative hypothesis in part (c) using non-definitive language.
Response 1 is given no credit because the response does not compare the p-value to any reasonable value of alpha, makes a correct decision, but uses definitive language (“there is an association”) when stating the conclusion in terms of the alternative hypothesis, in context.
Response 2 is given partial credit because the response correctly compares the p-value to alpha, but then contradicts the decision to reject H0 by saying “do not have convincing evidence,” before stating the conclusion in terms of the alternative hypothesis, in non-definitive language, in context.
Response 3 is given full credit because the response correctly compares the p-value to an alpha level, makes a correct decision and states the conclusion in terms of the alternative hypothesis, in non-definitive language, in context. The response does not explicitly need to state a decision about the null hypothesis.
Response 4 is given partial credit if the response reversed the hypotheses in part (c), because the response does not compare the p-value to alpha, but then states a correct decision and states the conclusion in terms of the alternative hypothesis (that they stated in part (c)--they already lost credit for the mistake once) in non-definitive language, in context. The response does not explicitly need to state a decision about the null hypothesis.
Response 5 is given partial credit because the response correctly compares the p-value to alpha by stating it is “very small,” makes a correct decision, but uses definitive language (“prove”) when stating the conclusion in terms of the alternative, in context.
Response 6 is given partial credit because the response sufficiently compares the p-value to alpha, makes a correct decision, but then states the conclusion in terms of the null hypothesis, in non-definitive language, in context, not the alternative hypothesis.
Response 7 is given partial credit because the response correctly compares the p-value to a common alpha level and makes a correct decision about the null hypothesis. The response does not state a conclusion in terms of the alternative hypothesis, in non-definitive language, in context.
Response 8 is given full credit because the response sufficiently compares the p-value to alpha (even though a value isn’t stated), makes a correct decision, and states a conclusion in terms of the alternative hypotheses, in non-definitive language, in context. The response does not explicitly need to state a decision about the null hypothesis.
Teaching tips:
Practice writing complete conclusions to significance tests including explicit comparison of the p-value to a significance level, making a correct decision, and stating a conclusion, in context, in terms of the alternative hypothesis in non-definitive language.
Students should not restate the null hypothesis in the conclusion.
Students should not attempt to state the interpretation of the p-value unless specifically asked to.
Students should be consistent between their decision about the null hypothesis and their conclusion (i.e. reject H0, there is convincing evidence of the alternative).
After added up all of the Es, Ps and Is, some responses will fall between two scores (like 2.5). In those cases, look at the entire response holistically, to decide whether to lower the score or raise the score a half point. Review the entire response to look for things that contribute to or take away from the overall quality of the response and thorough communication.
For this question, in parts (a) and (b), written thorough probability statements contribute to good communication and is considered a positive. In part (c), naming the test as a Chi-square Goodness-of-Fit test is a negative because it doesn’t demonstrate understanding that a two-way table doesn’t use that type of test. Naming the test something other than a chi-square test is a negative. Strong understanding of inference by writing correct hypotheses and writing a complete conclusion is a positive. Being inconsistent between comparison of the p-value to alpha and the decision and conclusion made is seen as a negative.
In general, if a response loses credit because of incomplete answers (i.e. stating the p-value but not comparing to alpha, or naming the test as just a chi-square test), the score is raised. If the response loses credit because of incorrect answers, the score is lowered. Also, look for parts where the response does something good, but isn’t enough to earn credit, to raise the score.
There were a lot of 3s and 4s on this question, which was fantastic! Because it is a multi-focus question, we saw many responses where students did great on the probability parts but poor on the inference parts, and vice versa, which was very interesting to see.



